trigonometry Proving trig identity $\tan (2x)−\tan (x)=\frac {\tan (x)} {\cos (2x)}$ Mathematics Stack ExchangeWe will use the following trigonometric formula to prove the formula for tan 2x tan (a b) = (tan a tan b)/ (1 tan a tan b) We have tan 2x = tan (x x) = (tan x tan x)/ (1 tan x tan x) = 2 tan x/ (1 tan 2 x) Hence, we have derived the tan 2x formula using the Use the double angle formulas to prove the identity csc 2 θ − cot 2 θ = tan θ \csc 2\theta \cot 2\theta = \tan \theta csc2θ−cot2θ = tanθ We have csc 2 θ − cot 2 θ = 1 sin 2 θ − cos 2 θ sin 2 θ = 1 − cos 2 θ sin 2 θ
What Is The Formula Of Tan2x Quora
Tan 2x identity proof
Tan 2x identity proof-To derive c), divide by y2 Or, we can derive both b) and c) from a) by dividing it first by cos 2θ and then by sin 2θ On dividing line 2) by cos 2θ, we have 1 tan 2θ = sec 2θ 1 cot 2θ = csc 2θ The three Pythagorean identities are thus equivalent to one another ProofThe inverse trigonometric functions are also called arcus functions or anti trigonometric functions These are the inverse functions of the trigonometric functions with suitably restricted domainsSpecifically, they are the inverse functions of the sine, cosine, tangent, cotangent, secant, and cosecant functions, and are used to obtain an angle from any of the angle's trigonometric
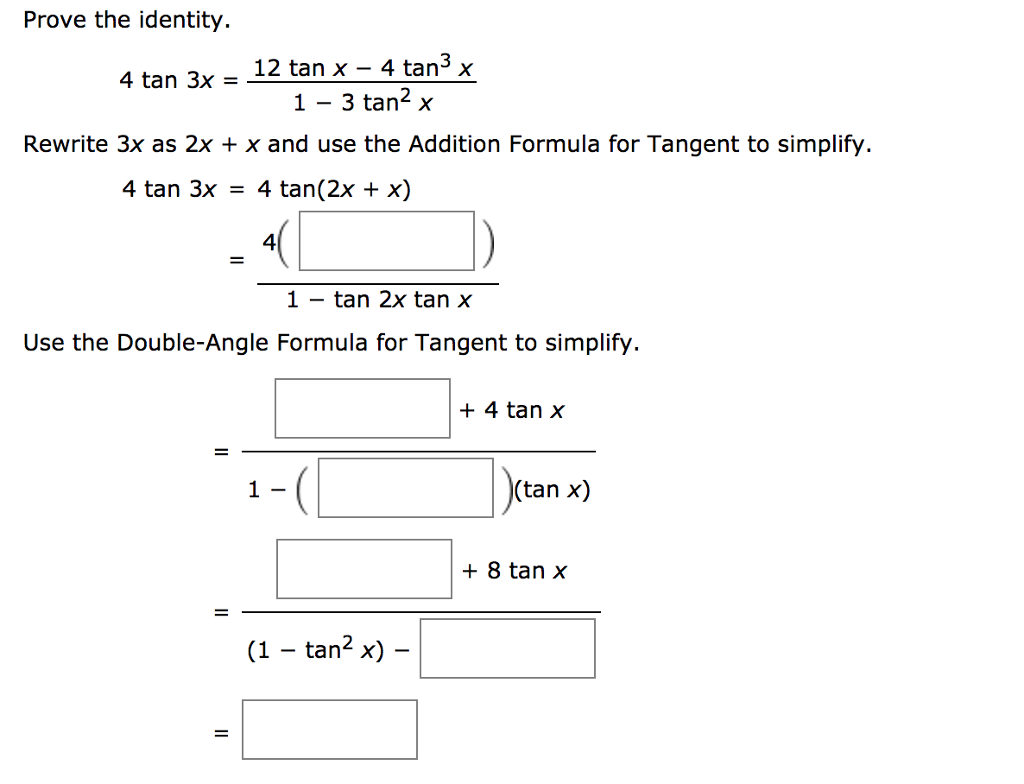



Solved Prove The Identity 4 Tan 3x 12 Tan X 4 Tan 3 Chegg Com
tan x = n tan y, sin x = m sin y then prove that m^2/n^21 = cos^2x trig Prove the identity sin(3 pi /2 x) sin(3pi/2 x) = 2cosx Maths complex numbers Find tan(3 theta) in terms of tan theta Use the formula tan (a b) = (tan a tan b)/1 tan a tan b) in two stepsTo determine the difference identity for tangent, use the fact that tan(−β) = −tanβ Example 1 Find the exact value of tan 75° Because 75° = 45° 30° Example 2 Verify that tan (180° − x) = −tan x Example 3 Verify that tan (180° x) = tan x Example 4 Verify that tan (360° − x) = − tan x The preceding three examples verify three formulas known as the reduction We have to prove that (tan x)^2 (sin x)^2 = (tan x)^2 * (sin x)^2 Start from the left hand side (tan x)^2 (sin x)^2 use tan x = sin x / cos x (sin x)^2/(cos x)^2 (sin x)^2
Stepbystep Solution Problem to solve s i n 2 x − t a n x = t a n x c o s 2 x sin2xtanx=tanxcos2x sin2x−tanx = tanxcos2x Choose the solving method Prove from LHS Prove from RHS Express everything into Sine and Cosine Learn how to solve trigonometric identities problems step by step online $\sin\left (2x\right)\frac {\sin\left (xI need to prove this identity tan^2xsin^2x = tan^2xsin^2x start with left side tan^2xsin^2x =(sin^2x/cos^2x)sin^2xTrigonometricidentitycalculator Prove tan^{2}(x) (1cot^{2}x) = sec^{2}x ar Related Symbolab blog posts Spinning The Unit Circle (Evaluating Trig Functions )
List of trigonometric identities 2 Trigonometric functions The primary trigonometric functions are the sine and cosine of an angle These are sometimes abbreviated sin(θ) andcos(θ), respectively, where θ is the angle, but the parentheses around the angle are often omitted, eg, sin θ andcos θ The tangent (tan) of an angle is the ratio of the sine to the cosineWe can prove the double angle identities using the sum formulas for sine and cosine From these formulas, we also have the following identities sin 2 x = 1 2 ( 1 − cos 2 x) cos 2 x = 1 2 ( 1 cos 2 x) sin x cos x = 1 2 ( sin 2 x) tan 2 x = 1 − cos 2 x 1 cos 2 xFor a proof like this, I like to start with the more difficultlooking side, and aim to end up with the expression on the other side Starting with the left hand side, the first thing to change is the sin2x, because the expression on the RHS only has terms of x, not 2xUsing the identity sin2x = 2sinxcosx, the expression becomes 2sinxcosx/(1 (tan 2 x))Next, we will change the tan 2 x to



What Is Integral Of Sin2x Tan2x Quora




Solved Prove The Identity 4 Tan 3x 12 Tan X 4 Tan 3 Chegg Com
In this video, we are going to derive the identity for the tangent of 3xThe identity tan(2x) has been explained in the following videohttps//youtube/IcU_mProve tan^2 (x)sin^2 (x)=tan^2 (x)sin^2 (x) Trigonometric Identities Solver Symbolab Identities Pythagorean Angle Sum/Difference Double Angle Multiple Angle Negative Angle Sum to Product Product to Sum Get an answer for 'Prove that tan^2x/(1tan^2x) = sin^2x' and find homework help for other Math questions at eNotes



Q35 A Prove That Tex Frac Tan 2x Tan X Tan 2x Tan X Sin 2x Tex Snapsolve




Solved Prove The Identity Cot 2x 1 Tan2 X 2 Tan X Chegg Com
To derive b), divide line (1) by x2; In this video, we are going to derive the identity for the tangent of 2xThe identity for tan(x y) has been explained in the following videohttps//youtubProof Half Angle Formula tan (x/2) Product to Sum Formula 1 Product to Sum Formula 2 Sum to Product Formula 1 Sum to Product Formula 2 Write sin (2x)cos3x as a Sum Write cos4xcos6x as a Product Prove cos^4 (x)sin^4 (x)=cos2x Prove sinxsin (5x)/ cosxcos (5x)=tan3x



How To Show That Math Tan 2 X Sec 2 X 1 Math Quora




上 Tan2x Identity ただの悪魔の画像
Prove sec^2xtan^2x=1 Trigonometric Identities Symbolab Identities Pythagorean Angle Sum/Difference Double Angle Multiple Angle Negative Angle Sum to Product Product to Sum Proof We will apply the following more fundamental trigonometric identities csc x = 1 sin x ( Reciprocal Identity) cos 2 x sin 2 x = 1 ( Pythagorean Identity) The proof is started from the lefthand side sin A csc A − sin 2 A = sin A ⋅ 1 sin A − sin 2 A = 1 − sin 2 A = cos 2 AThe more important identities You don't have to know all the identities off the top of your head But these you should Defining relations for tangent, cotangent, secant, and cosecant in terms of sine and cosine The Pythagorean formula for sines and cosines This is probably the most important trig identity




Proof Tan 2 1 Sec 2 Youtube




How Does One Verify Cos 2x Sin 2x 1 Tan 2x Cos 2x Socratic
It is proved the tan of sum of two angles is equal to the quotient of sum of tangents of both angles by the subtraction of products of tangents of both angles from one The expansion of tan of sum of two angles is called as angle sum identity for the tan function and also called as tan of compound angle identityGeneral Tan of Sum formula Now, let x=tan (A) and y=tan (B), so arctan (x)=A and arctan (y)=B, and then take the arctan of both sides, giving you So the lefthandside of the identity to be proven is The sum of arctans of reciprocals is pi/2, proving the identityNow divide numerator and denominator by cosAcosB to obtain the identity we wanted tan(AB) = tanAtanB 1−tanAtanB (16) We can get the identity for tan(A − B) by replacing B in (16) by −B and noting that tangent is an odd function tan(A−B) = tanA−tanB 1tanAtanB (17) 8 Summary There are many other identities that can be generated



3




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo
\tan \cot \csc \sec \alpha \beta \gamma \delta \zeta \eta \theta \iota \kappa \lambda \mu identity\\sin(2x) identity\\cos(2x) trigonometricidentitycalculator prove tan2x2tan2xsin^{2}x= sin2x he Related Symbolab blog posts Spinning The Unit Circle (Evaluating Trig Functions ) If you've ever taken a ferris wheel ride then youIntroduction to Tan double angle formula let's look at trigonometric formulae also called as the double angle formulae having double angles Derive Double Angle Formulae for Tan 2 Theta \(Tan 2x =\frac{2tan x}{1tan^{2}x} \) let's recall the addition formulaTrigonometric Identities are useful whenever trigonometric functions are involved in an expression or an equation Trigonometric Identities are true for every value of variables occurring on both sides of an equation Geometrically, these identities involve certain trigonometric functions (such as sine, cosine, tangent) of one or more angles Sine, cosine and tangent are the primary



How To Prove Cot X Tan X 2cosec 2x Cos 2x Quora




Verifying A Trigonometric Identity Cos X 1 Tan 2 X Cos X Sec X 1 Youtube
Question Prove each identity tan^2x sin^2x = tan^2xsin^2x Answer by greenestamps(9774) (Show Source) You can put this solution on YOUR website!• To obtain halfangle identity for tangent, we use the quotient identity and the half angle formulas for both cosine and sine tan x/2 = (sin x/2)/ (cos x/2) (quotient identity)Identities related to sin 2x, cos2x, tan 2x, sin3x, cos3x, and tan3x Sin 2x = Sin 2x = sin(2x)=2sin(x) cos(x) Sin(2x) = 2 * sin(x)cos(x) Proof To express Sine, the formula of "Angle Addition" can be used



70pmylqshcbm




Which Identity Needs To Be Used To Prove Tan Pi 2 X Cot X Brainly Com
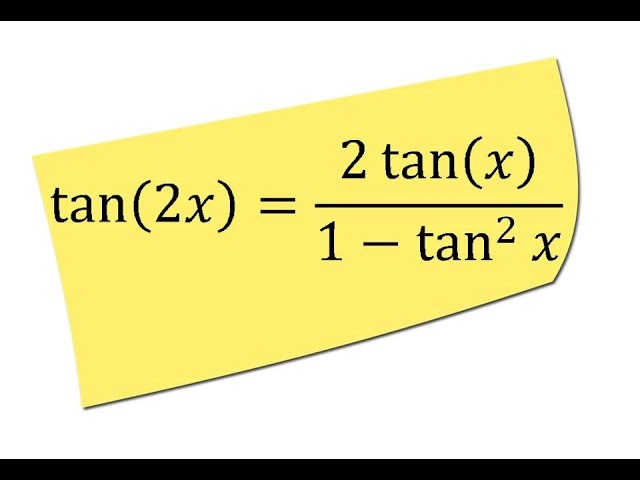
Prove each identity a) 1cos^2x=tan^2xcos^2x b) cos^2x 2sin^2x1 = sin^2x I also tried a question on my own tan^2x = (1 – cos^2x)/cos^2x RS= sin^2x/cos^2x I know that the Pythagorean for that is sin^2x cos^2x That's all I could do Calculus Okay so I have a question on my assignment that says You are given that tan(y) = xTan (x) is an odd function which is symmetric about its origin tan (2x) is a doubleangle trigonometric identity which takes the form of the ratio of sin (2x) to cos (2x) sin (2x) = 2 sin (x) cos (x) cos (2x) = (cos (x))^2 – (sin (x))^2 = 1 – 2 (sin (x))^2 = 2 (cos (x))^2 – 1 Proof 77K views View upvotes ·Now apply identity tan (ab)= (tan a tan b)/ (1tan atan b) tan2x can be solved by this method then by doing Tan (xx) and you will get tan2x=2tanx/ (1 tanxsquare) Tan3x=3tanx cube of tanx/1–3*square if tanx 7K views View upvotes ·



Tan2x Tan 2x Identity For Tan2x Proof Of Tan2x Identity Formula For Tan2x Youtube



Cos 2x Formula Derivation Examples What Is Cos 2a Formula
Answer to Prove the identity {1 tan^2 x} / {sin^2 x cos^2x} = sec^2 x By signing up, you'll get thousands of stepbystep solutions to your This is readily derived directly from the definition of the basic trigonometric functions sin and cos and Pythagoras's Theorem Divide both side by cos^2x and we get sin^2x/cos^2x cos^2x/cos^2x = 1/cos^2x tan^2x 1 = sec^2x tan^2x = sec^2x 1 Confirming that the result is an identity Answer linkSo sec^2 (x)=1tan^2 (x) This is one of the three Pythagorean identities in trigonometry, but if you don't recognize it, try converting to sines and cosines 1/cos^2 (x)=1sin^2 (x)/cos^2 (x) Now, multiply each term by cos^2 (x) to get 1=cos^2 (x) sin^2 (x




How Do You Verify 4tan 4 Tan 2x 3 Sec 2x 4tan 2x 3 Kinda Hard Please With All The Steps Thanks Socratic




Trigonometric Identity With Pythagorens Sec 2x Sin 2x Cos 2x Tan 2x Youtube
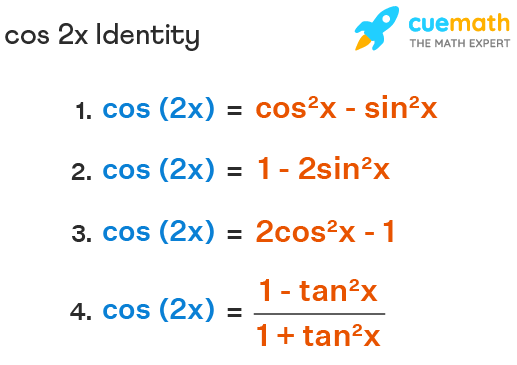
The subtraction of the tan squared of angle from secant squared of angle is equal to one and it is called as the Pythagorean identity of secant and tangent functions $\sec^2{\theta}\tan^2{\theta} \,=\, 1$ Popular forms The Pythagorean identity of secant and tan functions can also be written popularly in two other forms $\sec^2{x}\tan^2{x} \,=\, 1$Cos 2x is an important identity in trigonometry which can be expressed in different ways It can be expressed in terms of different trigonometric functions such as sine, cosine, and tangentCos 2x is one of the double angle trigonometric identities as the angle in consideration is a multiple of 2, that is, the double of xTrigonometric identities Intro to Pythagorean trigonometric identities Converting between trigonometric ratios example write all ratios in terms of sine Practice Evaluating expressions using basic trigonometric identities Trigonometric identity example proof involving sec, sin, and cos
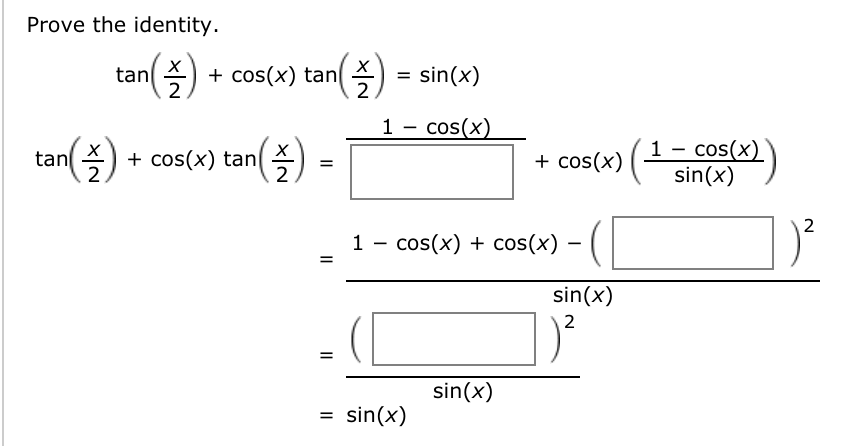



Solved Prove The Identity Cot 2x 1 Tan2x 2 Tan X Chegg Com




Precalculus Trigonometry Trig Identities 29 Of 57 Formula For Lowering Power Tan 2 X Youtube
Answer to Solved Prove the identity 1 cos(2x)/sin(2x) = tan(x) 1These identities are useful whenever expressions involving trigonometric functions need to be simplified An important application is the integration of nontrigonometric functions a common technique involves first using the substitution rule with a trigonometric function, and then simplifying the resulting integral with a trigonometric identityProve the trigonometric identity tan^2 (x)1=sec^2 (x) We can start with the identity sin 2 (x)cos 2 (x)=1 If we divide through the equation by cos 2 (x), we get sin 2 (x)/cos 2 (x) cos 2 (x)/cos 2 (x) = 1/cos 2 (x) If we look at the left hand side of the equation sin 2 (x)/cos 2 (x) is equal to tan 2 (x), and cos 2 (x)/cos 2 (x) is equal



View Question Trig Identities Need Help
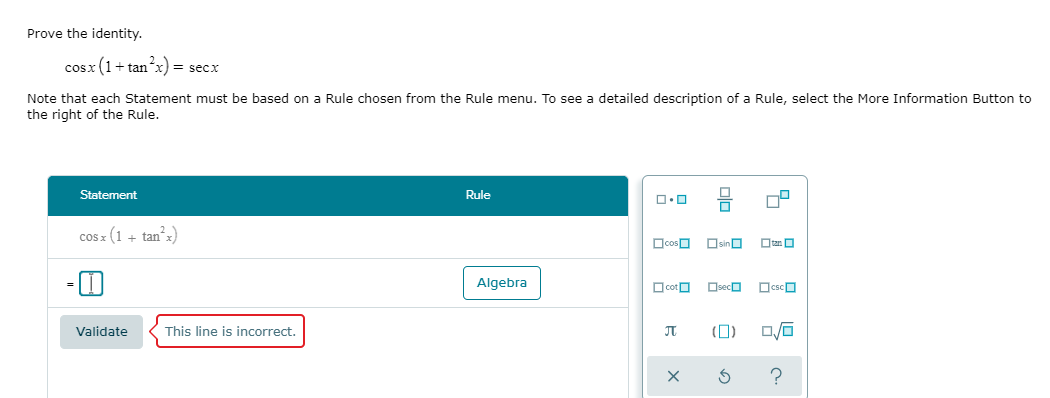



Solved Prove The Identity Cosx 1 Tan2x Secx Note That Chegg Com
A number of basic identities follow from the sum formulas for sine,cosine,and tangent The first category of identities involves doubleangle formulas Section 53 Group Exercise y = sin 2x 0, 2p, q by −3, 3, 1 sin 2x Z 2 sin x y = 2 sin x y = sin 2x tan 2u = 2 tan u We can prove the first two formulas in the box by working withQuestion I need to prove this identity tan^2xsin^2x = tan^2xsin^2x Answer by lwsshak3() (Show Source) You can put this solution on YOUR website!
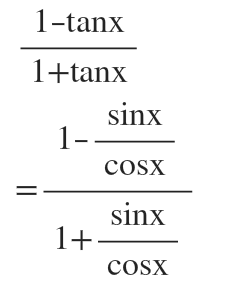



Answered Prove The Following Identity 1 Tan X Bartleby
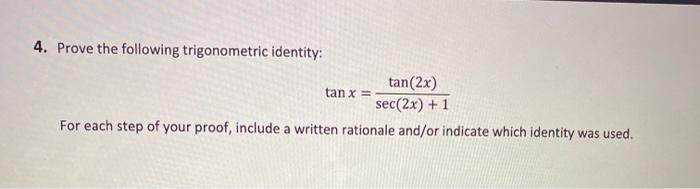



Solved 4 Prove The Following Trigonometric Identity Chegg Com
.JPG)



Every Day I M Calculatin I D3 Unit Q Pythagorean Identities




Trigonometric Identity Challenge Sin 2 Cos 2x Tan 2x Youtube




Double Angle Formulas Trigonometry Teachoo 2x 3x Formula Provi




Solved 16 0 3 Points Previous Answers Oscat1 9 3 155 My Chegg Com
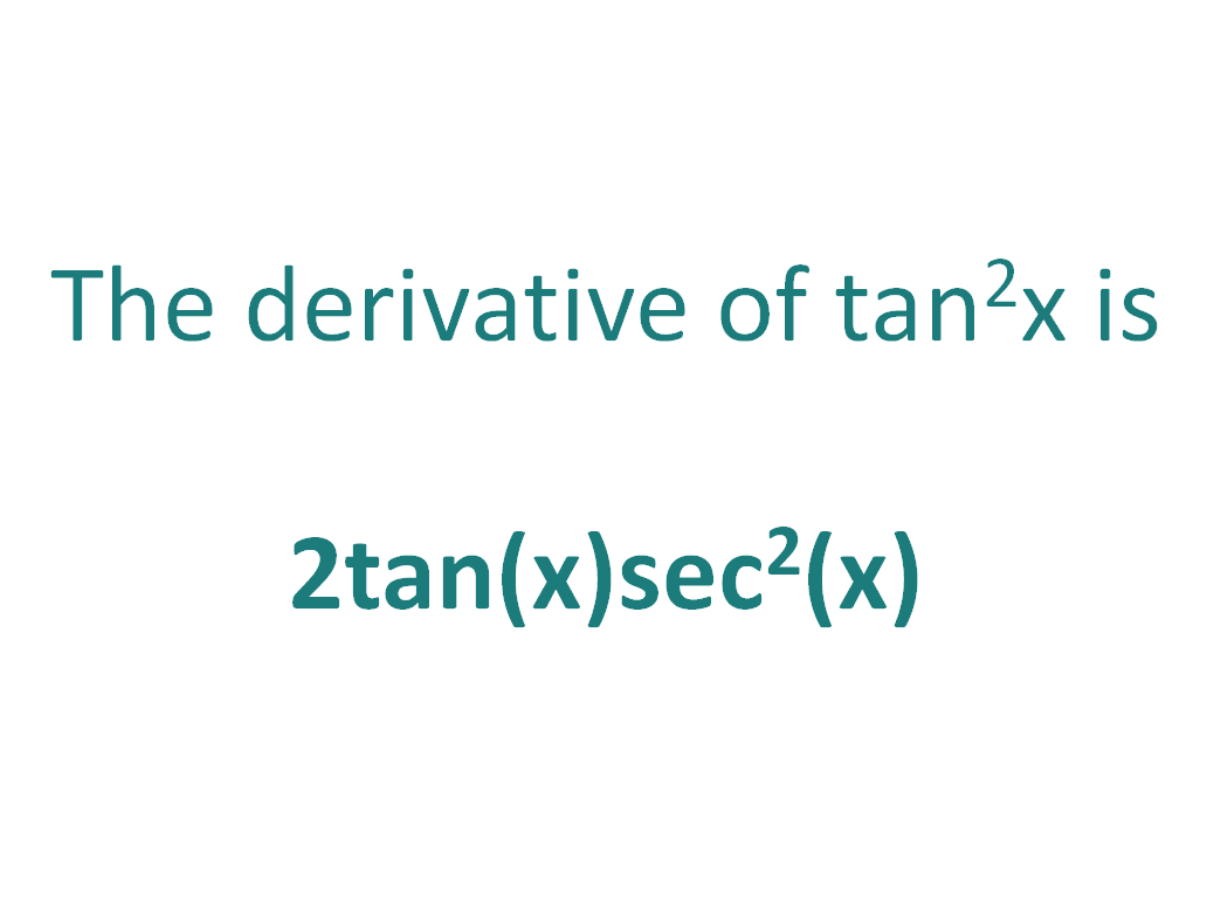



The Derivative Of Tan 2x Derivativeit
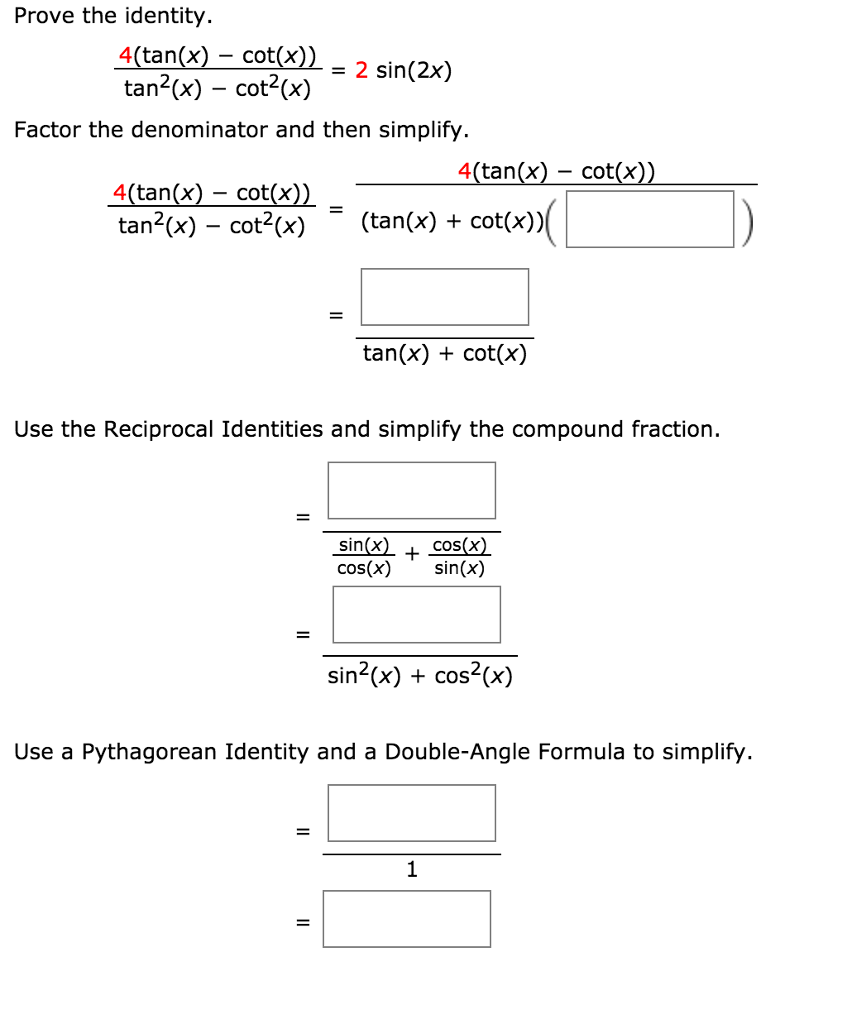



Solved Prove The Identity 4 Tan X Cot X Tan 2 X Chegg Com
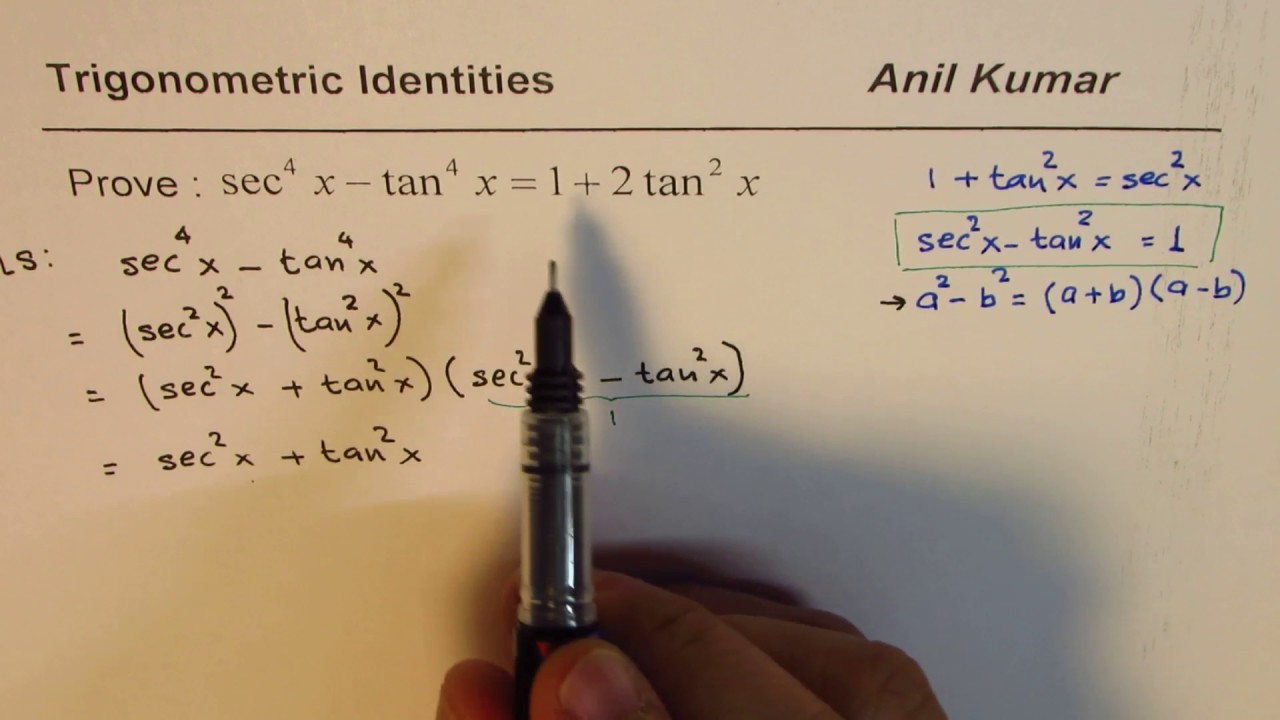



Trig Identity Sec 4x Tan 4x 1 2tan 2x Youtube



Tan2x 2tanx 1 Tan X Trigonometric Identity Solve Hindi Youtube




How Do You Prove The Identity Tan 2x Secx 1 1 Cosx Cosx Homeworklib




bestpictjcry Tan 2x Tan 2x




Trig Identity Sec2x Minus Tan2x T10 Youtube



Prove The Identity Secxcscx Tanx Cotx 2 Tan 2x Cot 2x1 Apply The Distributive Property2 Apply The Brainly Com



Trig Identities Prove Cot X Tan X 2tan 2x Physics Forums




How Many Can You Derive From First Principles Ppt Download




Sec 6x Tan 6x 1 2 Tan 2x Sec 2x Important Difficult Trigonometric Identity Youtube



How To Prove The Identity Tan2 X Sin2 X Tan2 X Sin2 X Quora



3




Solved Prove Or Disprove The Given Identity Sec 2 X Chegg Com
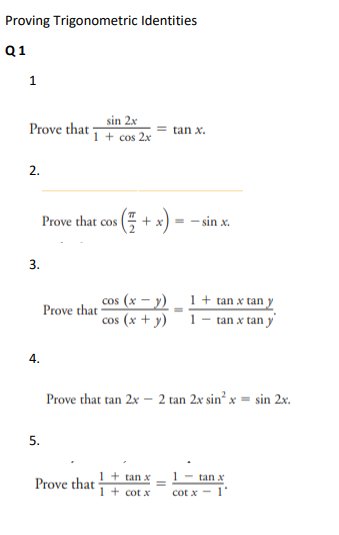



Solved Proving Trigonometric Identities Q1 Prove That Sin 2x Chegg Com
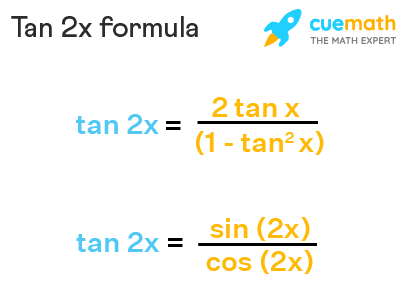



Tan 2x Formula What Is Tan 2x Formula Examples



Solved Prove The Following Trig Identities Course Hero



Solved Consider The Possible Identity Tan 2x Cos 2x 1 Cos 2x Sec 2x A State Any Non Permissible Values B Attempt To Verify Possible Identity Course Hero
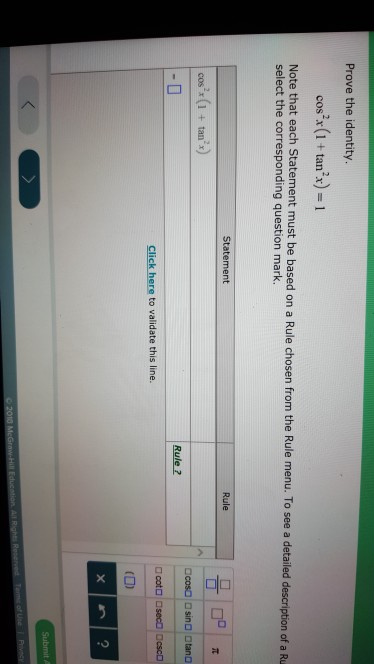



Solved Prove The Identity Cos 2x 1 Tan 2x 1 Note That Chegg Com




Tangent Half Angle Formula Wikipedia



上 Tan2x Identity ただの悪魔の画像




Tan2x 2tanx 1 Tan X Trigonometric Identity Solve Hindi Youtube



A Trig Identity




How Do You Prove Tan 2x Secx 1 1 Secx Socratic
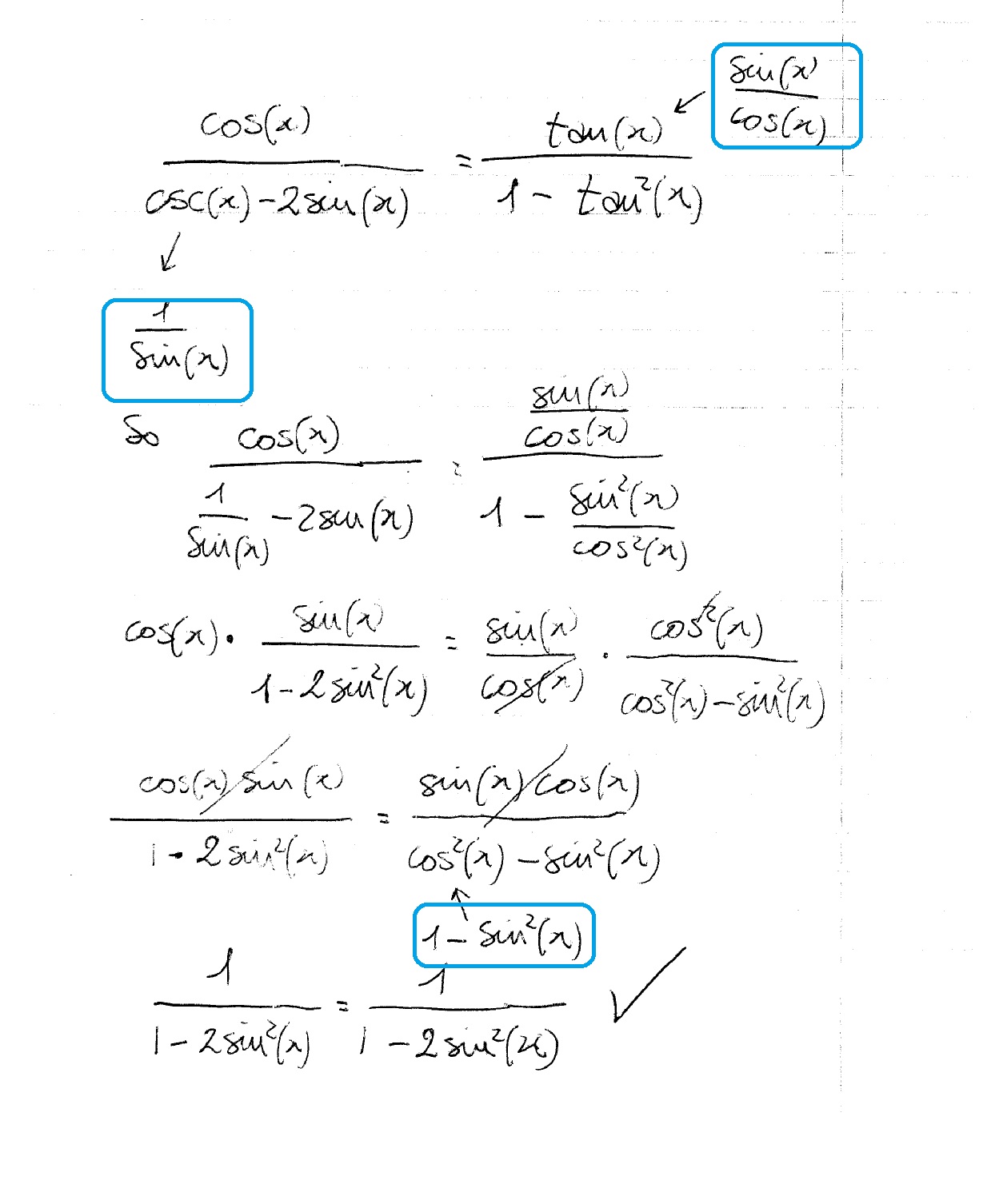



How Do You Prove Cosx Cscx 2sinx Tanx 1 Tan 2x Socratic



Solved Prove Trigonometric Identities Course Hero
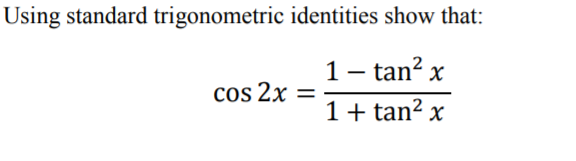



Solved Using Standard Trigonometric Identities Show That Chegg Com



What Is The Formula Of Tan2x Quora




Solved 2 Prove The Following Trig Identities A Prove Tan Chegg Com
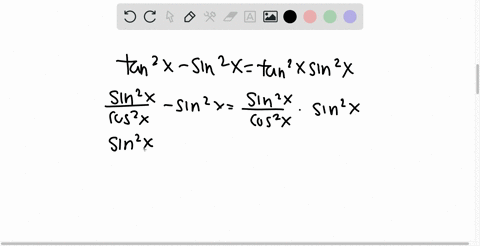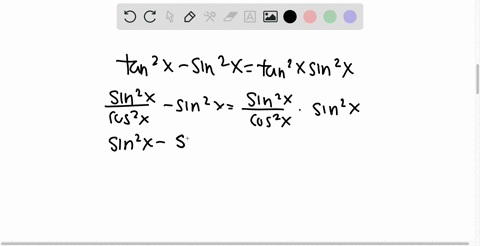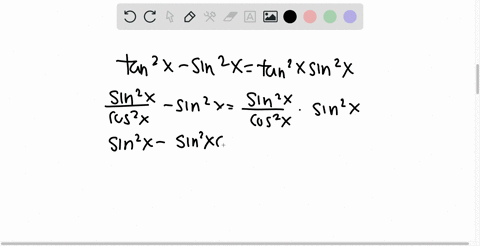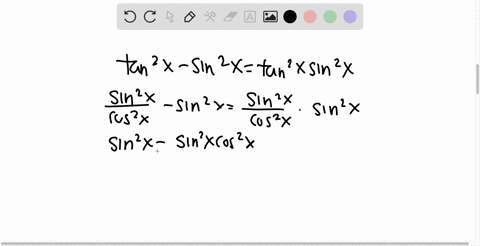



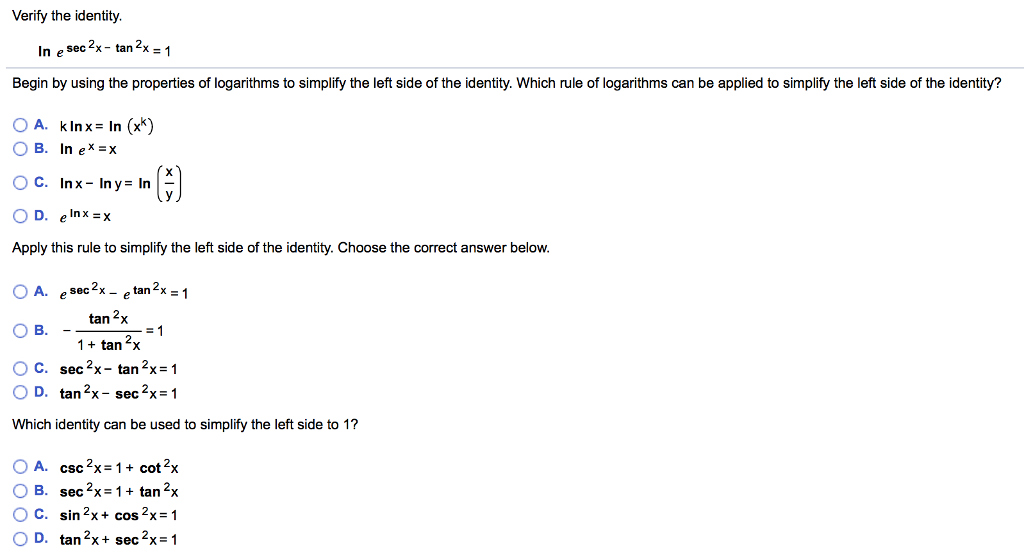
Solved Prove Each Identity Frac Tan 2 X Tan X Tan 2 X Tan X Sin 2 X



Tan2x 1 ただの悪魔の画像
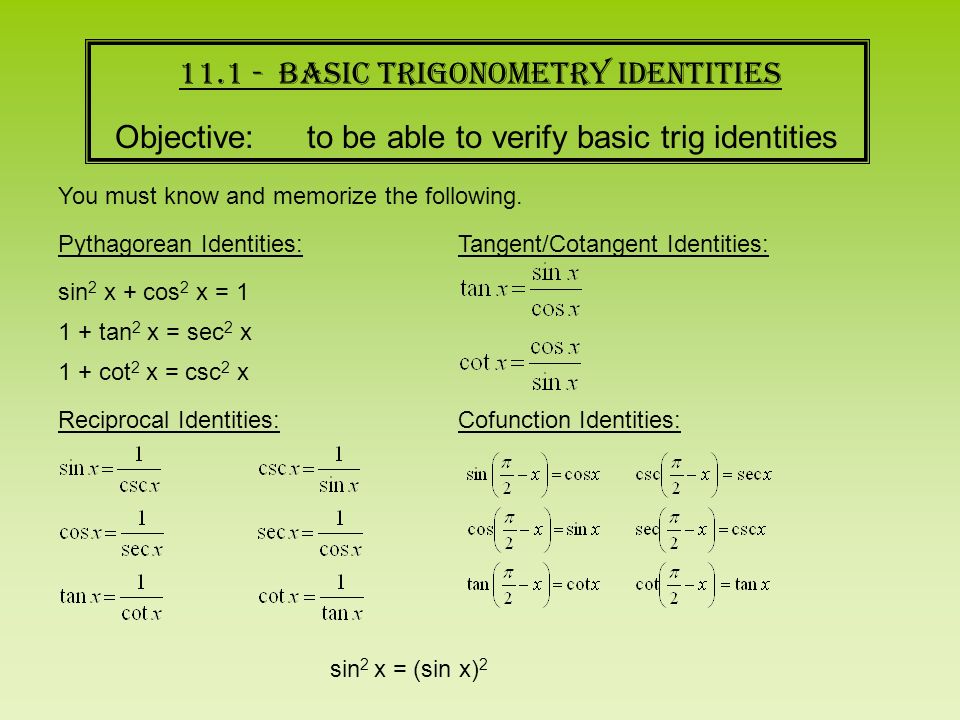



Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download
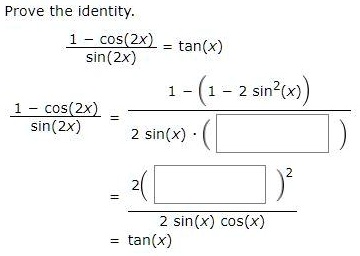



Solved Prove The Identity Cos 2x Tan X Sin 2x 1 1 2 Sin X Cos 2x Sin 2x 2 Sin X 2 Sin X Cos X Tan X



bestpictjcry Tan 2x Tan 2x



1



Bestmaths Online Proof 4
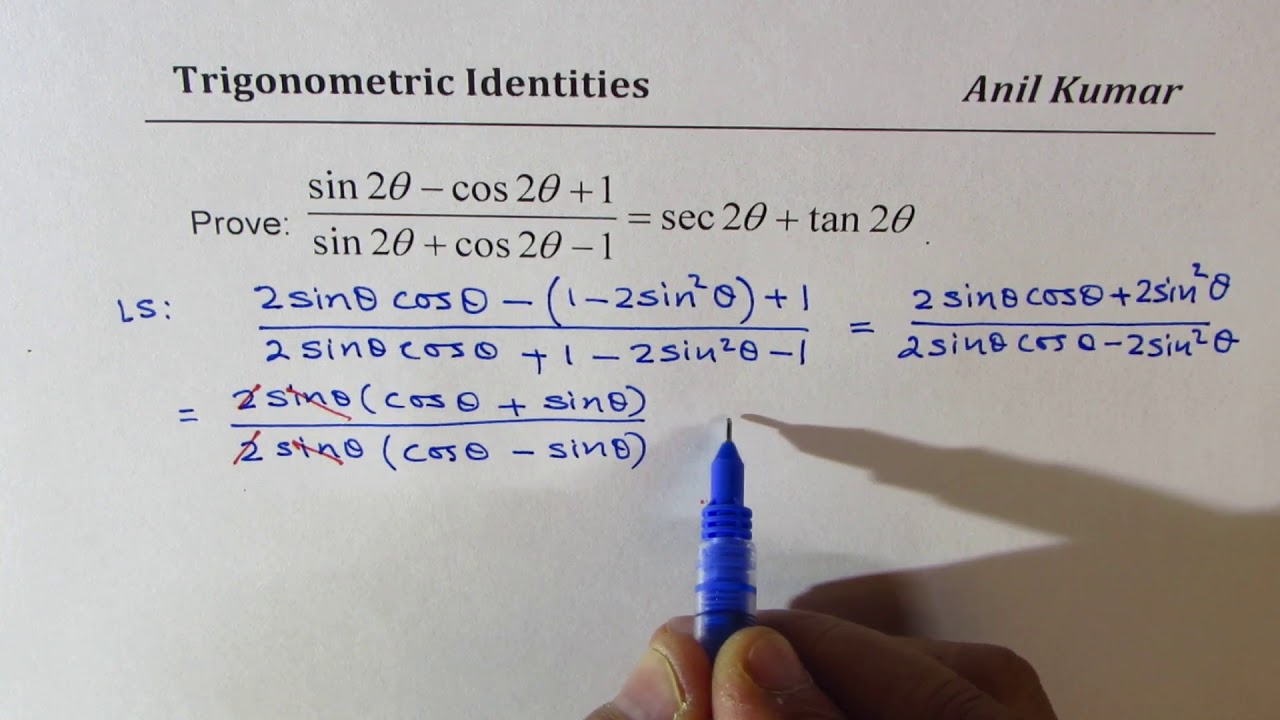



Trigonometric Identity In Double Angle Sin2x Cos2x 1 Sin2x Cos2x 1 Sec2x Tan2x Youtube




1 Tan 2x 1 Tan 2 X 1 2cos 2 X Youtube




bestpictjcry Tan 2x Tan 2x




How Do You Prove The Identities Cosx Secx Sinx Cscx Sec 2x Tan 2x Socratic



7 Proving Ids Trig Functions Identities



What Is The Formula Of Tan2x Quora




Tan2x Sec2x ただの悪魔の画像



What Is The Formula Of Tan2x Quora
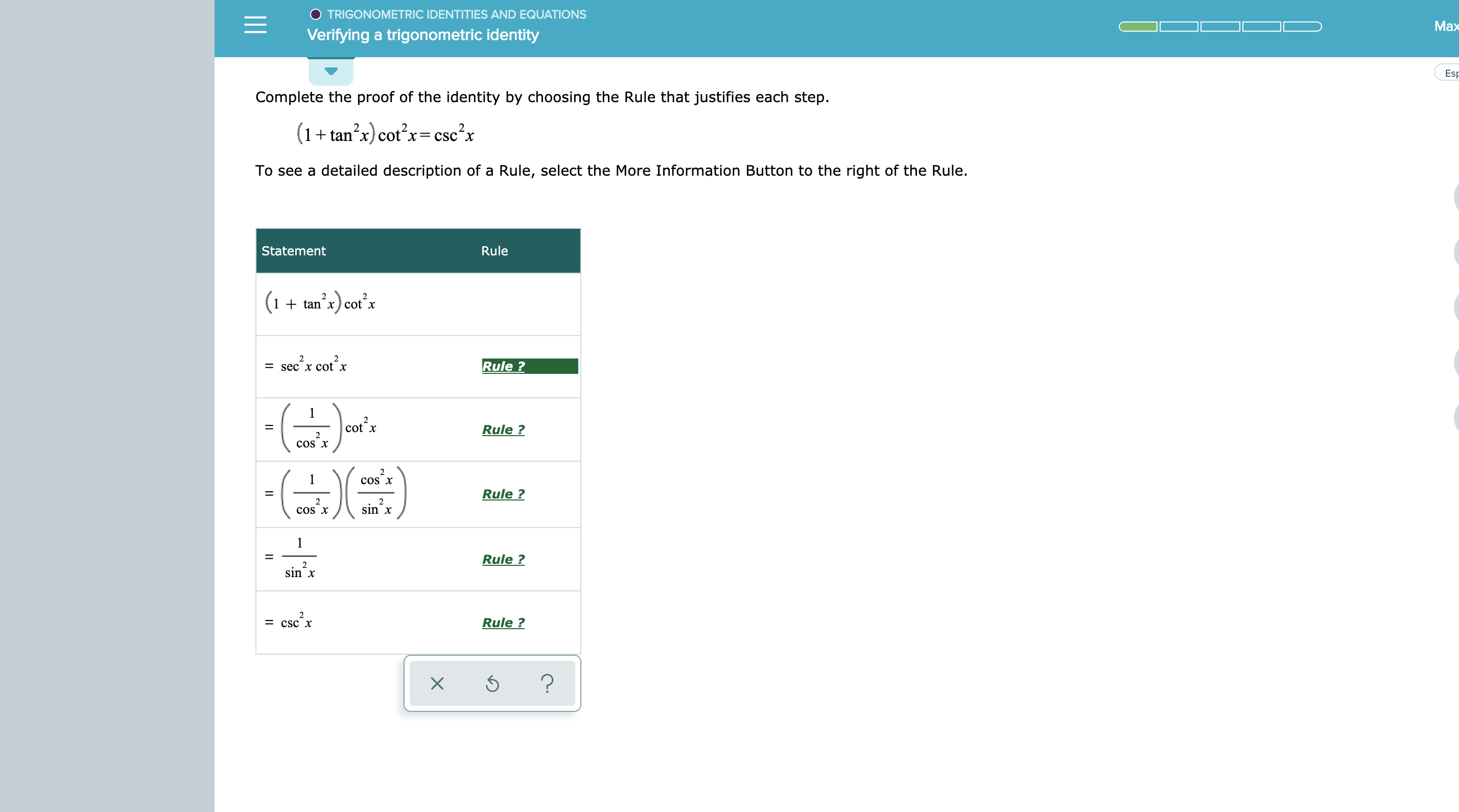



Answered Trigonometric Identities And Equations Bartleby



Tan2x 1 ただの悪魔の画像



1




Integrate Sec 2x Method 1
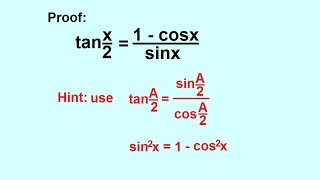



Lecture 129 Proof Half Angle Formula Tan X 2 Cosmolearning Mathematics



Prove Sin2x 2tanx 1 Tan 2x Socratic
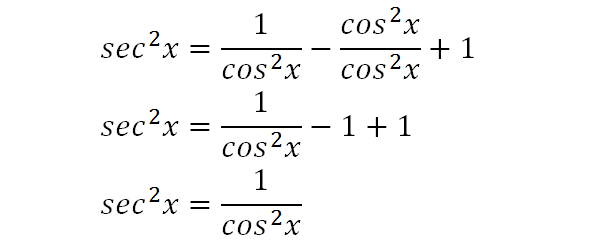



Integrate Sec 2x Method 2




Trigonometry Identity Tan 2 X 1 Sec 2 X Youtube
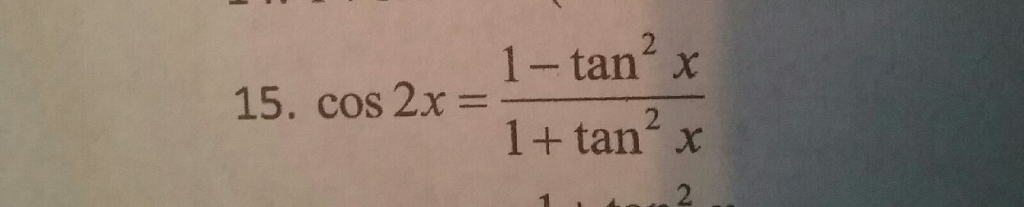



Solved Prove The Identity Cos 2x 1 Tan 2 X 1 Tan 2 X Chegg Com




How Do You Prove Tan 2x Secx 1 1 Secx Socratic



How To Solve Tan 2xsin 2x Tan 2x Sin 2x Trigonometry Trigonometric Identities Youtube




Ch 7 Trigonometric Identities And Equations Ppt Video Online Download




How Do You Prove The Identity Tan 2x Secx 1 1 Cosx Cosx Socratic




Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download




How Do You Simplify Sec 4x Tan 4x Sec 2x Tan 2x Socratic




How Do You Simplify 1 Tan 2 X 1 Tan 2 X Socratic
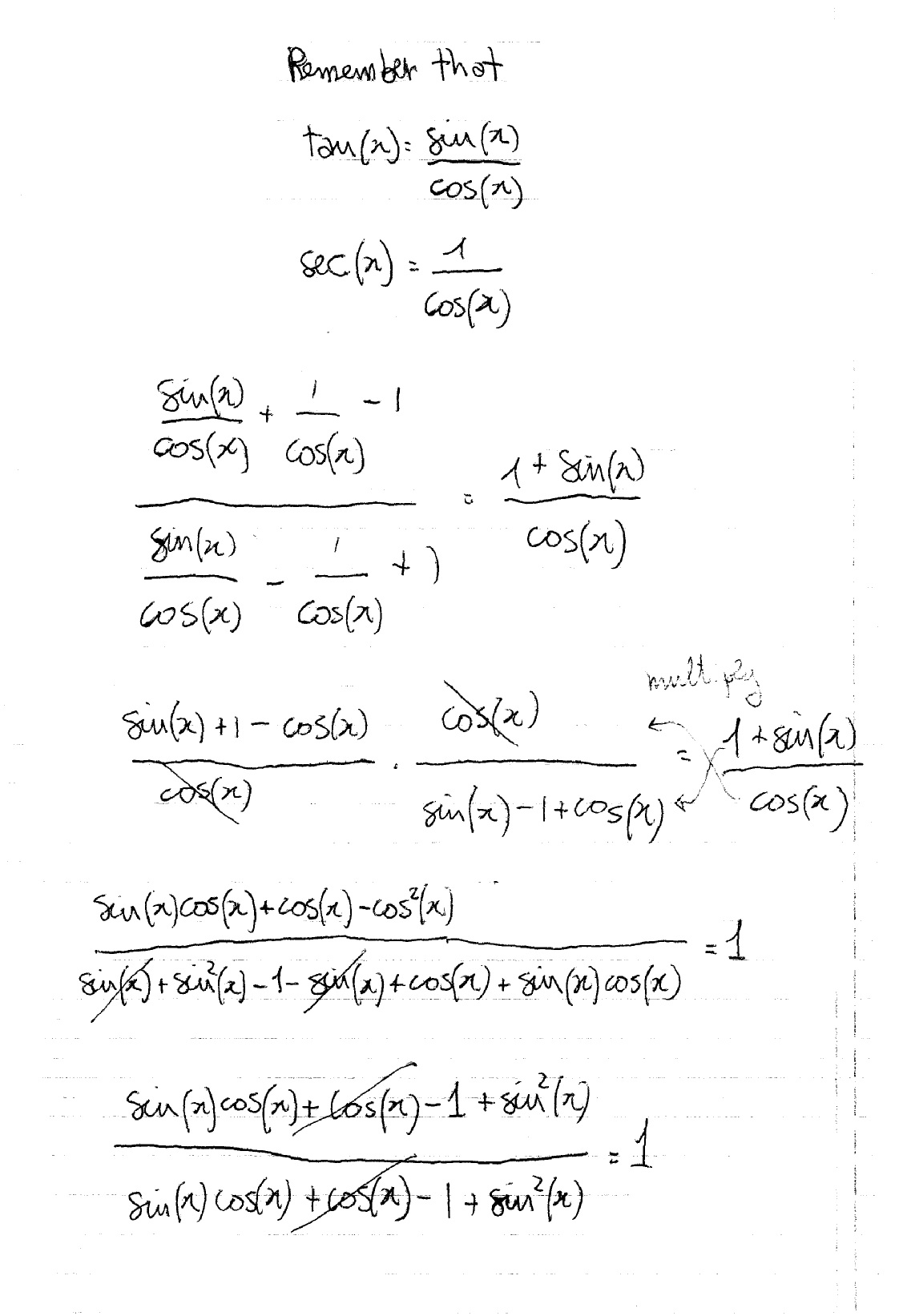



How Do You Prove That Tanx Secx 1 Tanx Secx 1 1 Sinx Cosx Socratic
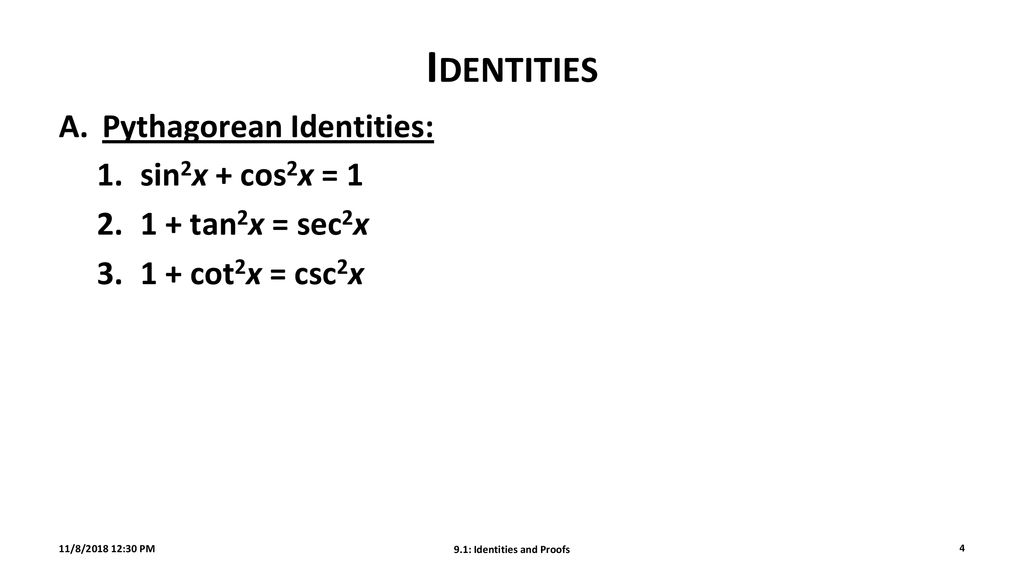



9 1 Identities And Proofs Ppt Download




Analytic Trig Ppt Video Online Download
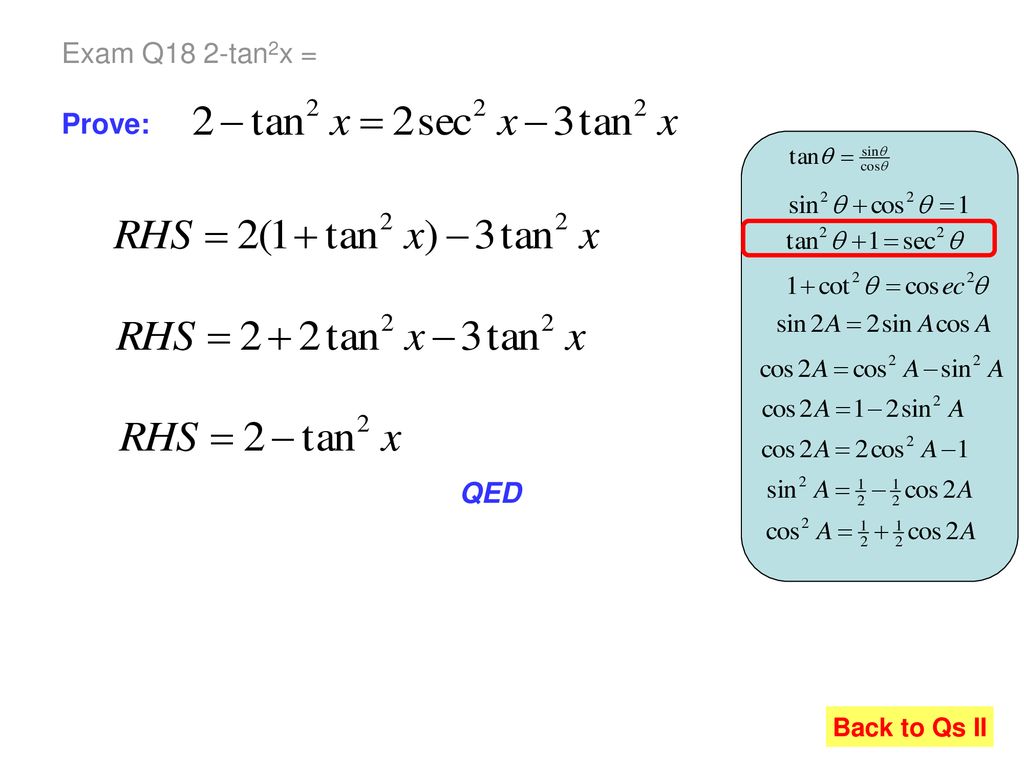



How Many Can You Derive From First Principles Ppt Download




How Do You Solve 1 Tan 2x 6 2sec 2x Socratic




Solved 2 Prove The Following Trig Identities A Prove Tan Chegg Com




Sin X 2 Tan X 2 1 Tan 2 X 2 Eraoco




Tan 2x Formula What Is Tan 2x Formula Examples



What Is The Formula Of Tan2x Quora




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo



Solved Prove The Following Trig Identity Sec 2 X 2secx Cosx Cos 2 X Tan 2 X Sin 2 X Course Hero




How Many Can You Derive From First Principles Ppt Download



What Is The Formula Of Tan2x Quora




Solved Complete The Proof Of The Identity By Choosing The Chegg Com




1 Tan 2x 1 Cos 2x Sin 2x 2sin 4x 1 Sin 2x Trigonometric Identities Mcr3u Youtube




Show That Tan 3xtan 2xtan X Tan 3x Tan 2x Tan X Orprove That Cos 2x Cos 2left X Frac Pi 3right Cos 2left X Frac Pi 3right Frac 3 2 Snapsolve



0 件のコメント:
コメントを投稿